Trigonometry, definition, uses of trigonometry and types of triangles.
Definition of trigonometry
The trigonometry is the branch of mathematics and geometry that is in charge of the study of triangles (a triangle is a polygon of 3 sides), this study is based in finding the relation between the sides and angles of a triangle, with the purpose of being able to find the sides and angles of a triangle only knowing a few variables.

Main characteristics of a triangle
The sides of a triangle can be measured in meters, centimeters etc, because it is a length measure, but to measure the angles of a triangle there are two options, measure them in radians or in degrees, usually in trigonometry the most common way to measure angles are in degrees.
Depending on the triangle we are studying, there are some characteristics that vary, but there are some characteristics that does not change no matter the type of triangle, this are constant, one of these is that the sum of the inside angles of a triangle will always be equals to 180, this is very useful when we have the value of two of the angels of the triangle and we want to find the third angle, like the sum of the three is 180, we just have to subtract to 180 the sum of the other two angles, this makes the process a little shorter.
Use of trigonometry in other branches
The study of triangles is applied in many subject more complex than geometry, the trigonometry is the base of a lot of physics and mathematics studies, for example, the vector topic is 100% based in the use of triangles and the vectors are use to represent forces, velocities, accelerations, etc. this means that a big part of physics is based in trigonometry.
Besides that, it has a big number of applications in robotic, each time we see a robot moving an arm or any other motion of the robot, this is almost always based in trigonometric calculations, like the degrees an angle has to extend so the robot reaches certain position, etc.

Difference between right and oblique triangles
In the study of triangles we can classify them in two types of triangles: the right triangles and the oblique triangles, the difference between the two is in their angles, a right triangle is a triangle that has a 90° angle, while we consider an oblique triangle to the triangles that does not have a right angle.
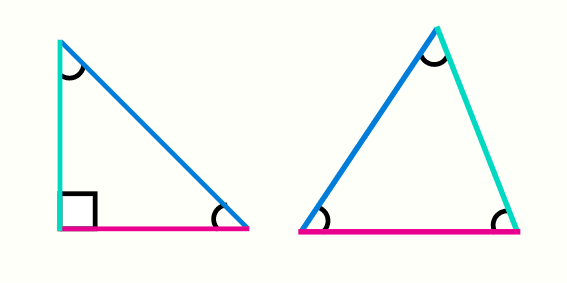
There are many ways of classifying a triangle, by its angles, sides or by a combination between sides and angles, but in mathematics and physics we are only going to classify the triangles in right and oblique triangles.
In trigonometry there are many theorems and formulas that have been developed to find the sides and angles of triangles, and there are specific formulas to find sides and angles of both types of triangles.
The theorems used in right triangles can only be used in right triangles, but the formulas used in oblique triangles can be used in right triangles as well, but it is easier to use the specific formula for each type of triangle.
Theorems, laws and formulas in trigonometry
Now we are going to present to you a short introduction of the theorems and laws in trigonometry.
Pythagorean theorem (right triangles)
The Pythagorean theorem allows us to find the length of a side if we know the other 2 sides. In this theorem we name the longest side of the triangle (the one that is the opposite side to the 90° angle) hypotenuse, while the other 2 triangles are called legs.
The Pythagorean theorem establishes that “the sum of the squared legs will be equals to the hypotenuse squared”,this theorem is only useful to find the missing side of a triangle, we cannot use it to find angles because the formula does not contain any angle, so this theorem is based only on sides.
- Pythagorean theorem
- C2 = A2 + B2
Trigonometric ratios (Right triangles)
The trigonometric ratios are another way to solve a right triangle, with the difference that this one can be use to find sides and angles of a triangle, these ratios are 3 formulas, these can be used such as we present them below or by clearing a variable.
- sen(Θ) = Cateto opuestoHipotenusa
- cos(Θ) = Cateto adyacenteHipotenusa
- tan(Θ) = Cateto opuestoCateto adyacente
Laws of sines and cosines (oblique triangles)
For the oblique triangles there are two equations, the sine law and the cosine law, with these two laws we have to analyze which one is more convenient to use in every situation, because both of them can be used to find sides and angles, but there is always a law that adapts better to each the situation.
- Law of the sines
- a⁄sen(A) = b⁄sen(B) = c⁄sen(C)
- o
- sen(A)⁄a = sen(B)⁄b = sen(C)⁄c
- Law of the cosines
- C2 = A2 + B2 - 2 * A * B * cos(c)
- B2 = A2 + C2 - 2 * A * C * cos(b)
- A2 = B2 + C2 - 2 * B * C * cos(a)
Related articles